نظریه آشوب
نظریه آشوب (به انگلیسی: Chaos Theory)، شاخه ای از ریاضیات است که بر روی مطالعه سامانههای پویای آشوبناک متمرکز است، سامانههایی که حالات بینظم و بدون ترتیبش ظاهراً تصادفی بوده اما در عمل تحت حاکمیت الگوها و قوانین قطعی پنهانیست که به شدت نسبت به شرایط اولیه حساسند.[1][2] نظریه آشوب شاخه ای بین رشته ایست که بیان میدارد: در سامانههای پیچیده با وجود تصادفی بودن ظاهری، الگوها، درون-پیوستگیها، حلقههای بازخوردی، تکرار، خودهمانندی، فراکتالها، و خود-سازماندهی وجود دارد.[3] اثر پروانهای، اصل زیربنایی نظریه آشوب بوده و به توصیف این پدیده میپردازد که چگونه تغییرات کوچک در یک سامانه قطعی و غیرخطی میتواند منجر به اختلافات بزرگی در یکی از حالات بعدی شود (یعنی وابستگی حساس بر روی شرایط آغازین).[4] استعاره ای از این رفتار، پروانه ای است که در تگزاس بال میزند قادر به ایجاد طوفانی در چین است.[5]
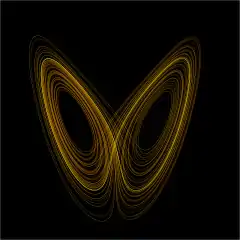

تغییرات کوچکی در شرایط اولیه، همچون تغییرات ناشی از خطاهای اندازهگیری ناشی از گرد کردن اعداد در محاسبات، میتواند باعث واگرایی گسترده خروجیهای چنین سامانههایی شده به گونه ای که پیشبینی بلند مدت رفتارشان را در حالت کلی غیرممکن میسازد.[6] با بوجودی که این سامانهها قطعی هستند، ممکن است چنین اتفاقی روی دهد. قطعی بودن به این معناست که رفتار آیندشان از سیر تکاملی منحصر بفردی تبعیت کرده[7] و بهطور کامل از طریق شرایط اولیه تعیین شده و هیچ نوع از عناصر تصادفی در تعیین آن دخیل نیست.[8] به بیانی دیگر، طبیعت قطعی این سامانهها، باعث پیشبینی پذیر شدنشان نیست.[9][10] به این نوع رفتار آشوب قطعی یا صرفاً آشوب میگویند. این نظریه توسط ادوارد لورنتس به این صورت خلاصه شد:[11]
آشوب: هنگامی که حال، آینده را تعیین میکند، اما حالِ تقریبی نتواند بهطور تقریبی آینده را تعیین کند.
رفتار آشوبناک در بسیاری از سامانههای طبیعی شامل این موارد وجود دارد: جریان سیال، بینظمیهای تپش قلب، آب و هوا و اقلیم.[12][13][7] همچنین این پدیده به صورت خود-به-خودی در برخی از سامانههایی با مؤلفههای مصنوعی نیز همچون بازار سهام و ترافیک جادهها نیز رخ میدهد.[14][3] این رفتار را میتوان از طریق تحلیل مدل ریاضیاتی، با کمک فنون تحلیلی چون نمودارهای بازگشتی و نگاشتهای پوانکاره مورد مطالعه قرار داد. نظریه آشوب در شاخههای متنوعی شامل این موارد کاربرد دارد: هواشناسی،[7] انسانشناسی،[15] جامعهشناسی،[16] علوم محیطی، علوم رایانه، مهندسی، اقتصاد، بومشناسی، مدیریت بحران همهگیری جهانی،[17][18] و فلسفه. این نظریه پایه شاخههای علمی چون سامانههای پویای پیچیده، نظریه مرز آشوب و فرایندهای خودسامانی را پی ریزی کرد.
مقدمه
یک سیستم جوی ساده را در نظر بگیرید. تابع برای تخمین دمای فردا از روی دمای امروز در دست است. اوربیت یک نقطهٔ تحت یک تابع از مجموعه اتفاقاتی است که در اثرِ تکرار تابع (دینامیک) برای آن نقطه میافتد. مثلاً، اُربیت نقطهٔ ۱ تحت این تابع این است که ۱ ابتدا ۳ و سپس ۵ و بعد ۷ و … میشود. مهمترین گونه مدارها نقطه ثابت است که هرگز تحت اجرای تابع تغییر نمیکند، ولی تابع ما چنین نقطهای ندارد. حال را در نظر بگیرید. این تابع ما را به دنیای آشوب میبرَد. به نظر میرسد مدارهای تمام نقاط به بینهایت میل میکنند. باید اشاره شود که نقاط پایانی هر بازهای روی این تابع ثابتاند. با اجرای تابع و ادامه دادن آن میبینیم که تمام نقاط داخل بازه به بینهایت میل میکنند، ولی حدود بازه همچنان متناهیاند. این رفتار آشوب گونه است. مثلث سرپینسکی و پوست مار کخ دو فراکتال یا برخال معروفاند. در مورد پوست مار کخ جالب این است که ناحیهٔ متناهی دارد، ولی پارامترش نامتناهی است. میتوان سطح خود تشابهی در فراکتالها را با مفهوم جدیدی از بُعد اندازهگیری کرد که مبتنی بر تعداد کپیهای مجموعههای خودمتشابه در فراکتال و میزان بزرگنمایی هر مجموعه است. یعنی بُعد فراکتالیِ یک مجموعه از تقسیم لگاریتم تعداد کپیها به لگاریتم بزرگنمایی به دست میآید. این مقدار برای مثلث سرپینسکی ۱٫۵۸۴ و برای پوست مار کخ ۱٫۲۶۱ است.
تاریخچه
این نظریه گسترش خود را بیشتر مدیون کارهای هانری پوانکاره، ادوارد لورنتس، بنوا مندلبروت و مایکل فیگنباوم است. پوانکاره اولین کسی بود که اثبات کرد مسئله سه جرم (به عنوان مثال، خورشید، زمین، ماه) مسئلهٔ آشوبی و غیرقابل حل است. شاخهٔ دیگر از نظریهٔ آشوب که در مکانیک کوانتومی به کار میرود، آشوب کوانتومی نام دارد. گفته میشود که پیر لاپلاس و عمر خیام قبل از پوانکاره، به این مسئله و پدیده پی برده بودند.
اولین آزمایش واقعی در زمینهٔ آشوب را یک هواشناس به نام ادوارد لورنتس انجام داد. در سال ۱۹۶۰، وی روی یک مسئلهٔ پیشبینی وضع هوا کار میکرد؛ و روی کامپیوترش ۱۲ معادله برای پیشبینی وضع هوا در نظر گرفته بود. این معادلهها وضعِ هوا را پیشبینی نمیکرد، ولی این برنامهٔ کامپیوتری بهطور نظری پیشبینی میکرد که هوا چگونه میتواند باشد. او میخواست دنبالهٔ مشخصی را دوباره ببیند. برای کاهش زمان، وی به جای شروع از اول، از وسط دنباله شروع کرد. او عددی را وارد کرد که دفعهٔ قبل از دنباله در دست داشت و کامپیوتر را برای پردازش رها نمود و رفت. وقتی یک ساعت بعد برگشت، دنباله به صورتی متفاوت از دفعهٔ قبل پیشرفت کرده بود. به جای حالت قبلی، الگوی جدید آن واگرا میشد و در آخر شکلی کاملاً به هم ریخته نسبت به اولی پیدا میکرد. بالاخره فهمید که مشکل کار کجاست. کامپیوتر تا ۶ رقم اعشار را در خود ذخیره میکرد و برای اینکه کاغذ کمتری مصرف کند، فقط تا ۳ رقم اعشار را برای خروجی در نظر گرفته بود. در الگوی اولیه، عدد بهدست آمده در اصل۵۰۶۱۲۷/۰ بود، ولی برای حالت بعدی فقط ۵۰۶/۰ را وارد کرد. براساس تمام ایدههای آن زمان، این دنباله باید شبیه یا خیلی نزدیک به حالت اولیه میشد. رقمهای پنجم و ششم، که برای بعضی از روشها غیرقابل اندازهگیری هستند، نمیتوانند تأثیر زیادی روی خروجی داشته باشند. لورنز این باور را رد کرد. این اثر به عنوان اثر پروانهای شناخته شد. مقدار تفاوت بین نقاط شروع دو نمودار آنقدر کم است، که به اندازهٔ بال زدن یک پروانه میتواند باشد؛ بال زدن یک پروانه تغییر بسیار اندکی در وضعیت اتمسفر ایجاد میکند. در طول یک دوره، اتمسفر از حالتی که باید میبود، عملاً دور میشود. به همین دلیل، در طول یک دوره، یک گردباد که قرار بود سواحل اندونزی را تخریب کند، هیچ وقت اتفاق نمیافتد یا ممکن است گردبادی رخ دهد که اصلاً قرار نبود اتفاق بیفتد. این پدیده به عنوانِ حساسیت بالا به شرایط اولیه نیز شناخته شدهاست. *
جستارهای وابسته
منابع
- "The Definitive Glossary of Higher Mathematical Jargon — Chaos". Math Vault. 2019-08-01. Retrieved 2019-11-24.
- "chaos theory | Definition & Facts". Encyclopedia Britannica. Retrieved 2019-11-24.
- "What is Chaos Theory? – Fractal Foundation". Retrieved 2019-11-24.
- Weisstein, Eric W. "Chaos". mathworld.wolfram.com. Retrieved 2019-11-24.
- Boeing, Geoff. "Chaos Theory and the Logistic Map". Retrieved 2020-05-17.
- Kellert, Stephen H. (1993). In the Wake of Chaos: Unpredictable Order in Dynamical Systems. University of Chicago Press. p. 32. ISBN 978-0-226-42976-2.
- Bishop, Robert (2017), "Chaos", in Zalta, Edward N., The Stanford Encyclopedia of Philosophy (Spring 2017 ed.), Metaphysics Research Lab, Stanford University, retrieved 2019-11-24
- Kellert 1993, p. 56
- Kellert 1993, p. 62
- Werndl, Charlotte (2009). "What are the New Implications of Chaos for Unpredictability?". The British Journal for the Philosophy of Science. 60 (1): 195–220. arXiv:1310.1576. doi:10.1093/bjps/axn053. S2CID 354849.
- Danforth, Christopher M. (April 2013). "Chaos in an Atmosphere Hanging on a Wall". Mathematics of Planet Earth 2013. Retrieved 12 June 2018.
- Lorenz, Edward N. (1963). "Deterministic non-periodic flow". Journal of the Atmospheric Sciences. 20 (2): 130–141. Bibcode:1963JAtS...20..130L. doi:10.1175/1520-0469(1963)020<0130:DNF>2.0.CO;2.
- Ivancevic, Vladimir G.; Tijana T. Ivancevic (2008). Complex nonlinearity: chaos, phase transitions, topology change, and path integrals. Springer. ISBN 978-3-540-79356-4.
- Safonov, Leonid A.; Tomer, Elad; Strygin, Vadim V.; Ashkenazy, Yosef; Havlin, Shlomo (2002). "Multifractal chaotic attractors in a system of delay-differential equations modeling road traffic". Chaos: An Interdisciplinary Journal of Nonlinear Science. 12 (4): 1006–1014. Bibcode:2002Chaos..12.1006S. doi:10.1063/1.1507903. ISSN 1054-1500. PMID 12779624.
- Mosko M.S. , Damon F.H. (Eds.) (2005). On the order of chaos. Social anthropology and the science of chaos. Oxford: Berghahn Books.
- Hubler, A (1989). "Adaptive control of chaotic systems". Swiss Physical Society. Helvetica Physica Acta 62: 339–342.
- Piotrowski, Chris. "Covid-19 Pandemic and Chaos Theory: Applications based on a Bibliometric Analysis". researchgate.net. Retrieved 2020-05-13.
- Weinberger, David (2019). Everyday Chaos - Technology, Complexity, and How We're Thriving in a New World of Possibility. Harvard Business Review Press. ISBN 978-1-63369-396-8.
برای مطالعه بیشتر
مقالات
- Sharkovskii, A.N. (1964). "Co-existence of cycles of a continuous mapping of the line into itself". Ukrainian Math. J. 16: 61–71.
- Li, T.Y.; Yorke, J.A. (1975). "Period Three Implies Chaos" (PDF). American Mathematical Monthly. 82 (10): 985–92. Bibcode:1975AmMM...82..985L. CiteSeerX 10.1.1.329.5038. doi:10.2307/2318254. JSTOR 2318254. Archived from the original (PDF) on 29 December 2009. Retrieved 21 April 2021.
- Alemansour, Hamed; Miandoab, Ehsan Maani; Pishkenari, Hossein Nejat (March 2017). "Effect of size on the chaotic behavior of nano resonators". Communications in Nonlinear Science and Numerical Simulation. 44: 495–505. Bibcode:2017CNSNS..44..495A. doi:10.1016/j.cnsns.2016.09.010.
- Crutchfield; Tucker; Morrison; J.D. Farmer; Packard; N.H.; Shaw; R.S (December 1986). "Chaos". Scientific American. 255 (6): 38–49 (bibliography p.136). Bibcode:1986SciAm.255d..38T. doi:10.1038/scientificamerican1286-46. Online version (Note: the volume and page citation cited for the online text differ from that cited here. The citation here is from a photocopy, which is consistent with other citations found online that don't provide article views. The online content is identical to the hardcopy text. Citation variations are related to country of publication).
- Kolyada, S.F. (2004). "Li-Yorke sensitivity and other concepts of chaos". Ukrainian Math. J. 56 (8): 1242–57. doi:10.1007/s11253-005-0055-4. S2CID 207251437.
- Day, R.H.; Pavlov, O.V. (2004). "Computing Economic Chaos". Computational Economics. 23 (4): 289–301. doi:10.1023/B:CSEM.0000026787.81469.1f. S2CID 119972392. SSRN 806124.
- Strelioff, C.; Hübler, A. (2006). "Medium-Term Prediction of Chaos" (PDF). Phys. Rev. Lett. 96 (4): 044101. Bibcode:2006PhRvL..96d4101S. doi:10.1103/PhysRevLett.96.044101. PMID 16486826. 044101. Archived from the original (PDF) on 2013-04-26.
- Hübler, A.; Foster, G.; Phelps, K. (2007). "Managing Chaos: Thinking out of the Box" (PDF). Complexity. 12 (3): 10–13. Bibcode:2007Cmplx..12c..10H. doi:10.1002/cplx.20159. Archived from the original (PDF) on 2012-10-30. Retrieved 2011-07-17.
- Motter, Adilson E.; Campbell, David K. (2013). "Chaos at 50". Physics Today. 66 (5): 27. arXiv:1306.5777. Bibcode:2013PhT....66e..27M. doi:10.1063/PT.3.1977. S2CID 54005470.
کتب درسی
- Alligood, K.T.; Sauer, T.; Yorke, J.A. (1997). Chaos: an introduction to dynamical systems. Springer-Verlag. ISBN 978-0-387-94677-1.
- Baker, G. L. (1996). Chaos, Scattering and Statistical Mechanics. Cambridge University Press. ISBN 978-0-521-39511-3.
- Badii, R.; Politi A. (1997). Complexity: hierarchical structures and scaling in physics. Cambridge University Press. ISBN 978-0-521-66385-4.
- Bunde; Havlin, Shlomo, eds. (1996). Fractals and Disordered Systems. Springer. ISBN 978-3642848704. and Bunde; Havlin, Shlomo, eds. (1994). Fractals in Science. Springer. ISBN 978-3-540-56220-7.
- Collet, Pierre, and Eckmann, Jean-Pierre (1980). Iterated Maps on the Interval as Dynamical Systems. Birkhauser. ISBN 978-0-8176-4926-5.
- Devaney, Robert L. (2003). An Introduction to Chaotic Dynamical Systems (2nd ed.). Westview Press. ISBN 978-0-8133-4085-2.
- Robinson, Clark (1995). Dynamical systems: Stability, symbolic dynamics, and chaos. CRC Press. ISBN 0-8493-8493-1.
- Feldman, D. P. (2012). Chaos and Fractals: An Elementary Introduction. Oxford University Press. ISBN 978-0-19-956644-0. Archived from the original on 31 December 2019. Retrieved 21 April 2021.
- Gollub, J. P.; Baker, G. L. (1996). Chaotic dynamics. Cambridge University Press. ISBN 978-0-521-47685-0.
- Guckenheimer, John; Holmes, Philip (1983). Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Springer-Verlag. ISBN 978-0-387-90819-9.
- Gulick, Denny (1992). Encounters with Chaos. McGraw-Hill. ISBN 978-0-07-025203-5.
- Gutzwiller, Martin (1990). Chaos in Classical and Quantum Mechanics. Springer-Verlag. ISBN 978-0-387-97173-5.
- Hoover, William Graham (2001) [1999]. Time Reversibility, Computer Simulation, and Chaos. World Scientific. ISBN 978-981-02-4073-8.
- Kautz, Richard (2011). Chaos: The Science of Predictable Random Motion. Oxford University Press. ISBN 978-0-19-959458-0.
- Kiel, L. Douglas; Elliott, Euel W. (1997). Chaos Theory in the Social Sciences. Perseus Publishing. ISBN 978-0-472-08472-2.
- Moon, Francis (1990). Chaotic and Fractal Dynamics. Springer-Verlag. ISBN 978-0-471-54571-2.
- Ott, Edward (2002). Chaos in Dynamical Systems. Cambridge University Press. ISBN 978-0-521-01084-9.
- Strogatz, Steven (2000). Nonlinear Dynamics and Chaos. Perseus Publishing. ISBN 978-0-7382-0453-6.
- Sprott, Julien Clinton (2003). Chaos and Time-Series Analysis. Oxford University Press. ISBN 978-0-19-850840-3.
- Tél, Tamás; Gruiz, Márton (2006). Chaotic dynamics: An introduction based on classical mechanics. Cambridge University Press. ISBN 978-0-521-83912-9.
- Teschl, Gerald (2012). Ordinary Differential Equations and Dynamical Systems. Providence: American Mathematical Society. ISBN 978-0-8218-8328-0.
- Thompson JM, Stewart HB (2001). Nonlinear Dynamics And Chaos. John Wiley and Sons Ltd. ISBN 978-0-471-87645-8.
- Tufillaro; Reilly (1992). An experimental approach to nonlinear dynamics and chaos. American Journal of Physics. 61. Addison-Wesley. p. 958. Bibcode:1993AmJPh..61..958T. doi:10.1119/1.17380. ISBN 978-0-201-55441-0.
- Wiggins, Stephen (2003). Introduction to Applied Dynamical Systems and Chaos. Springer. ISBN 978-0-387-00177-7.
- Zaslavsky, George M. (2005). Hamiltonian Chaos and Fractional Dynamics. Oxford University Press. ISBN 978-0-19-852604-9.
آثار نیمه-فنی و عرفی
- Christophe Letellier, Chaos in Nature, World Scientific Publishing Company, 2012, شابک ۹۷۸−۹۸۱−۴۳۷۴−۴۲−۲ .
- Abraham, Ralph; et al. (2000). Abraham, Ralph H.; Ueda, Yoshisuke, eds. The Chaos Avant-Garde: Memoirs of the Early Days of Chaos Theory. World Scientific Series on Nonlinear Science Series A. 39. World Scientific. Bibcode:2000cagm.book.....A. doi:10.1142/4510. ISBN 978-981-238-647-2.
- Barnsley, Michael F. (2000). Fractals Everywhere. Morgan Kaufmann. ISBN 978-0-12-079069-2.
- Bird, Richard J. (2003). Chaos and Life: Complexity and Order in Evolution and Thought. Columbia University Press. ISBN 978-0-231-12662-5.
- John Briggs and David Peat, Turbulent Mirror: : An Illustrated Guide to Chaos Theory and the Science of Wholeness, Harper Perennial 1990, 224 pp.
- John Briggs and David Peat, Seven Life Lessons of Chaos: Spiritual Wisdom from the Science of Change, Harper Perennial 2000, 224 pp.
- Cunningham, Lawrence A. (1994). "From Random Walks to Chaotic Crashes: The Linear Genealogy of the Efficient Capital Market Hypothesis". George Washington Law Review. 62: 546.
- Predrag Cvitanović, Universality in Chaos, Adam Hilger 1989, 648 pp.
- Leon Glass and Michael C. Mackey, From Clocks to Chaos: The Rhythms of Life, Princeton University Press 1988, 272 pp.
- James Gleick, Chaos: Making a New Science, New York: Penguin, 1988. 368 pp.
- John Gribbin. Deep Simplicity. Penguin Press Science. Penguin Books.
- L Douglas Kiel, Euel W Elliott (ed.), Chaos Theory in the Social Sciences: Foundations and Applications, University of Michigan Press, 1997, 360 pp.
- Arvind Kumar, Chaos, Fractals and Self-Organisation; New Perspectives on Complexity in Nature , National Book Trust, 2003.
- Hans Lauwerier, Fractals, Princeton University Press, 1991.
- Edward Lorenz, The Essence of Chaos, University of Washington Press, 1996.
- Marshall, Alan (2002). The Unity of Nature - Wholeness and Disintegration in Ecology and Science. doi:10.1142/9781860949548. ISBN 9781860949548.
- David Peak and Michael Frame, Chaos Under Control: The Art and Science of Complexity, Freeman, 1994.
- Heinz-Otto Peitgen and Dietmar Saupe (Eds.), The Science of Fractal Images, Springer 1988, 312 pp.
- Clifford A. Pickover, Computers, Pattern, Chaos, and Beauty: Graphics from an Unseen World , St Martins Pr 1991.
- Clifford A. Pickover, Chaos in Wonderland: Visual Adventures in a Fractal World, St Martins Pr 1994.
- Ilya Prigogine and Isabelle Stengers, Order Out of Chaos, Bantam 1984.
- Peitgen, Heinz-Otto; Richter, Peter H. (1986). The Beauty of Fractals. doi:10.1007/978-3-642-61717-1. ISBN 978-3-642-61719-5.
- David Ruelle, Chance and Chaos, Princeton University Press 1993.
- Ivars Peterson, Newton's Clock: Chaos in the Solar System, Freeman, 1993.
- Ian Roulstone; John Norbury (2013). Invisible in the Storm: the role of mathematics in understanding weather. Princeton University Press. ISBN 978-0691152721.
- Ruelle, D. (1989). Chaotic Evolution and Strange Attractors. doi:10.1017/CBO9780511608773. ISBN 9780521362726.
- Manfred Schroeder, Fractals, Chaos, and Power Laws, Freeman, 1991.
- Smith, Peter (1998). Explaining Chaos. doi:10.1017/CBO9780511554544. ISBN 9780511554544.
- Ian Stewart, Does God Play Dice?: The Mathematics of Chaos , Blackwell Publishers, 1990.
- Steven Strogatz, Sync: The emerging science of spontaneous order, Hyperion, 2003.
- Yoshisuke Ueda, The Road To Chaos, Aerial Pr, 1993.
- M. Mitchell Waldrop, Complexity : The Emerging Science at the Edge of Order and Chaos, Simon & Schuster, 1992.
- Antonio Sawaya, Financial Time Series Analysis : Chaos and Neurodynamics Approach, Lambert, 2012.
پیوند به بیرون
- "Chaos", Encyclopedia of Mathematics, EMS Press, 2001 [1994]
- Nonlinear Dynamics Research Group with Animations in Flash
- The Chaos group at the University of Maryland
- The Chaos Hypertextbook. An introductory primer on chaos and fractals
- ChaosBook.org An advanced graduate textbook on chaos (no fractals)
- Society for Chaos Theory in Psychology & Life Sciences
- Nonlinear Dynamics Research Group at CSDC, Florence Italy
- Interactive live chaotic pendulum experiment, allows users to interact and sample data from a real working damped driven chaotic pendulum
- Nonlinear dynamics: how science comprehends chaos, talk presented by Sunny Auyang, 1998.
- Nonlinear Dynamics. Models of bifurcation and chaos by Elmer G. Wiens
- Gleick's Chaos (excerpt)
- Systems Analysis, Modelling and Prediction Group at the University of Oxford
- A page about the Mackey-Glass equation
- High Anxieties — The Mathematics of Chaos (2008) BBC documentary directed by David Malone
- The chaos theory of evolution – article published in Newscientist featuring similarities of evolution and non-linear systems including fractal nature of life and chaos.
- Jos Leys, Étienne Ghys et Aurélien Alvarez, Chaos, A Mathematical Adventure. Nine films about dynamical systems, the butterfly effect and chaos theory, intended for a wide audience.
- "Chaos Theory", BBC Radio 4 discussion with Susan Greenfield, David Papineau & Neil Johnson (In Our Time, May 16, 2002)


.jpg.webp)
